Page 25 - 新思维数学学生用书5 样章

2 2D shape and pattern

Think like a mathematician

Explore these questions by drawing triangles and diagrams to show what is possible

and what is impossible.
a What type of triangle can have a right angle?

b What type of triangle can have two right angles?
c What type of triangle can have three right angles?

d Investigate the number of acute angles and obtuse angles the different types of

triangles can have. Write sentences to describe the angle properties of different
types of triangles.

• You are generalising when you describe which triangles are possible with each
number of right angles.

• You are convincing when you show which angles are possible and which are

impossible in different triangles.

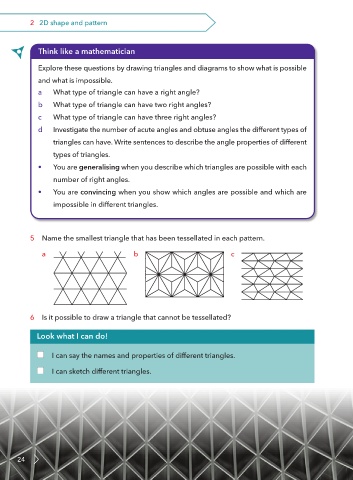
5 Name the smallest triangle that has been tessellated in each pattern.

a b c

6 Is it possible to draw a triangle that cannot be tessellated?

Look what I can do!

I can say the names and properties of different triangles.

I can sketch different triangles.

24